Last active
November 17, 2022 15:54
-
-
Save mutoo/5617691 to your computer and use it in GitHub Desktop.
a very fast algorithm for getting the circumcircle from a triangle - http://www.exaflop.org/docs/cgafaq/cga1.html#Subject 1.04
This file contains hidden or bidirectional Unicode text that may be interpreted or compiled differently than what appears below. To review, open the file in an editor that reveals hidden Unicode characters.
Learn more about bidirectional Unicode characters
| function circumcircle(a, b, c) { | |
| this.a = a | |
| this.b = b | |
| this.c = c | |
| var A = b.x - a.x, | |
| B = b.y - a.y, | |
| C = c.x - a.x, | |
| D = c.y - a.y, | |
| E = A * (a.x + b.x) + B * (a.y + b.y), | |
| F = C * (a.x + c.x) + D * (a.y + c.y), | |
| G = 2 * (A * (c.y - b.y) - B * (c.x - b.x)), | |
| minx, miny, dx, dy | |
| /* If the points of the triangle are collinear, then just find the | |
| * extremes and use the midpoint as the center of the circumcircle. */ | |
| if(Math.abs(G) < 0.000001) { | |
| minx = Math.min(a.x, b.x, c.x) | |
| miny = Math.min(a.y, b.y, c.y) | |
| dx = (Math.max(a.x, b.x, c.x) - minx) * 0.5 | |
| dy = (Math.max(a.y, b.y, c.y) - miny) * 0.5 | |
| this.x = minx + dx | |
| this.y = miny + dy | |
| this.r = dx * dx + dy * dy | |
| } | |
| else { | |
| this.x = (D*E - B*F) / G | |
| this.y = (A*F - C*E) / G | |
| dx = this.x - a.x | |
| dy = this.y - a.y | |
| this.r = dx * dx + dy * dy | |
| } | |
| } |
Sign up for free
to join this conversation on GitHub.
Already have an account?
Sign in to comment
Depending on your circumstances you can do it with vectors. The intersection of at least two rays, each passing through the midpoint of a side of the triangle and perpendicular to that side, is the circumcenter. Proof seen here: https://flashman.neocities.org/Courses/Circumcenter.html
In this image below the (segments of) rays are M, N and P, each perpendicular to the side that they pass through the midpoint of:
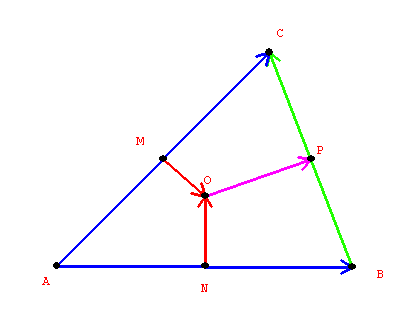
The circumcenter is O, equidistant to the 3 vertices. In some cases it can be outside of the triangle.